Statistical properties of kinetic-scale magnetic holes in terrestrial space

1.   Introduction

Magnetic holes (MHs) are structures characterized by a significant magnetic depression, and have been widely observed in space plasmas. These structures were first identified in the solar wind (Turner et al., 1977), and were subsequently observed frequently in the planetary magnetosheath (e.g., Cattaneo et al., 1998; Balikhin et al., 2009; Tsurutani et al., 2011; Yao ST et al., 2017), in the magnetotail (e.g., Ge YS et al., 2011; Yao ST et al., 2016; Zhang XJ et al., 2017), in the magnetospheric cusp (Shi QQ et al., 2009; Jasinski et al., 2017), and even in the magnetosphere of comets (Russell et al., 1987; Plaschke et al., 2018). They have been identified under various names according to their generation mechanisms and property features, for example, “magnetic dips”, “magnetic decreases”, “mirror modes”, and “solitary waves” (e.g., Song P et al., 1992, 1994; Baumgärtel, 1999; Lucek et al., 1999; Horbury et al., 2004; Stasiewicz, 2004; Tsurutani et al., 2011; Yao ST et al., 2018b; Tian AM et al., 2018, 2020; Zhang L et al., 2018; Treumann and Baumjohann, 2019; Wang GQ et al., 2020a; Kitamura et al., 2020). Of these terms, the first two describe the observable phenomenon, while the last two emphasize the generation mechanism and nature.

Kinetic-scale magnetic holes (KSMHs), structures with a significant magnetic depression within the proton gyroradius scale, have attracted much attention in recent years. These structures were first identified in the Earth’s magnetotail by Ge YS et al. (2011) and Sun WJ et al. (2012). Various possible generation mechanisms have been proposed, including mirror modes, tearing structures, solitary waves, and ballooning/interchange instabilities (Balikhin et al., 2012; Ji XF et al., 2014; Sundberg et al., 2015; Yao ST et al., 2016; Li ZY et al., 2016; Shustov et al., 2019). Zhang XJ et al. (2017) found that KSMHs can modulate electron cyclotron harmonic waves, and are important for the coupling of Earth’s magnetosphere and ionosphere. Using the high temporal resolution data collected onboard NASA’s Magnetospheric MultiScale (MMS) mission (Burch et al., 2016), KSMHs associated with electron vortices were observed in the magnetosheath by Yao ST et al. (2017). The electron vortex was found to be due to the combination of electron diamagnetic motion and E x B motion (Yao ST et al., 2017; Li JH et al., 2020a). Inside the KSMHs, the flux of lower energy electrons was decreased while that of higher energy electrons was increased. This is evidence of electron acceleration inside the KSMHs. A schematic of the KSMHs is shown in Figure 1, accompanied by a typical event observed in the magnetosheath. The scales, boundaries (Liu H et al., 2019a, b), and topologies (Liu YY et al., 2020) of these KSMHs were studied further by applying an innovative particle sounding technique (Zong Q-G et al., 2004), and second-order Taylor expansion (SOTE) method (Liu YY et al., 2019). The propagation, contraction, and expansion of these KSMHs were recently investigated by Yao ST et al. (2020a) based on multi-spacecraft analysis methods (Shi QQ et al., 2005, 2006, 2019; Xiao T et al., 2015; Rezeau et al., 2018; Wang MM et al., 2020). Li JH et al. (2020b) further analyzed the particle behavior and associated distribution functions during the kinetic evolution of the KSMHs. Similar events have also been reported in simulations (Haynes et al., 2015; Roytershteyn et al., 2015) and observations (Huang SY et al., 2017a, b). These structures, including recently reported kinetic-scale flux ropes (Huang SY et al., 2016; Matsui et al., 2019; Sun WJ et al., 2019; Wang SM et al., 2019; Yao ST et al., 2020b) and kinetic-scale magnetic dips and peaks (Hellinger and Štverák, 2018; Stawarz et al., 2018; Yao ST et al., 2018a; Hoilijoki et al., 2019), are new types of coherent structures found in turbulent plasmas, and play important roles in the cascade of turbulence from ion to electron scales (e.g., Huang J et al., 2019; Lucek et al., 2005; Karimabadi et al., 2014; Sahraoui et al., 2020; Shang WS et al., 2020). Yao ST et al. (2019a) found that these KSMHs are coupled with electron cyclotron waves, electrostatic solitary waves, and whistler mode waves (Li Z et al., 2019). The observations revealed that electron beams and temperature anisotropy within KSMHs provide free energy to generate such waves, implying that the KSMHs transfer energy at kinetic scales in turbulent magnetosheath plasmas. The coupling of KSMHs with whistler mode waves, including wave formation, dispersion, and growth rates, was also studied by Huang SY et al. (2018, 2019). Zhong ZH et al. (2019) reported a KSMH event associated with strong energy dissipation near the active X line at the dayside magnetopause. They suggested that the KSMH probably provided an additional channel for energy dissipation besides that of the electron diffusion region. The MMS mission also revealed new features of KSMHs observed in the Earth’s magnetotail and solar wind. For example, electron dynamics (diamagnetic and ${\boldsymbol E}\times {\boldsymbol B}$ drift) in KSMHs were investigated by Gershman et al. (2016) and Goodrich et al. (2016). The distributions of KSMHs exhibited dawn–dusk asymmetry in the magnetotail, with ~72% of the KSMHs accompanied by substorms (Huang SY et al., 2019). Train-like KSMHs were observed in the solar wind, and clear evidence indicated that they were electron mirror mode structures (Yao ST et al., 2019b). The generation, geometry, and particle behaviors of the solar wind KSMHs were further studied by Wang GQ et al. (2020b, c, d).

Figure  1.  A schematic of a KSMH and a typical KSMH observed in the magnetosheath by MMS.

DownLoad: Full-Size Img PowerPoint

As indicated above, KSMHs with their generation mechanisms, effects, and impacts have been extensively investigated because of their importance and potential roles in the space environment. However, there remain difficult open questions regarding their structure source, generation environment, and spatio–temporal characteristics. For example, although KSMHs can be observed in both solar wind and the magnetosheath, to date it has not been clear whether some fraction of KSMHs are generated locally in the magnetosheath or whether they are all carried into the magnetosheath by the solar wind. What are their spatio–temporal characteristics and what are the underlying physical processes that generate and maintain their structure? In this article, we investigate these questions by carrying out a statistical study of the KSMHs observed by the MMS mission. The data set and event selection are introduced in Section 2. The distributions of the KSMHs’s occurrence rate, amplitude, and temporal and spatial scales are shown in Section 3. A discussion and conclusions are presented in Section 4.

2.   Data and Event Selection

Although the MMS burst mode data were widely and well used in previous studies, only ~2%–4% of the time per day of burst mode data can be downloaded to the ground (Fuselier et al., 2016). The lower time resolution (fast and survey mode) MMS data from September 2015 to March 2020 are used in this study, since these data cover most of the orbit. The time resolution of survey mode magnetic field data from the Fluxgate Magnetometer (FGM) instrument is 16 Hz (Russell et al., 2016), and of the fast mode ion data from the Fast Plasma Investigation (FPI) instrument is 0.22 Hz (Pollock et al., 2016). The Geocentric Solar Ecliptic (GSE) coordinate system is used throughout this article.

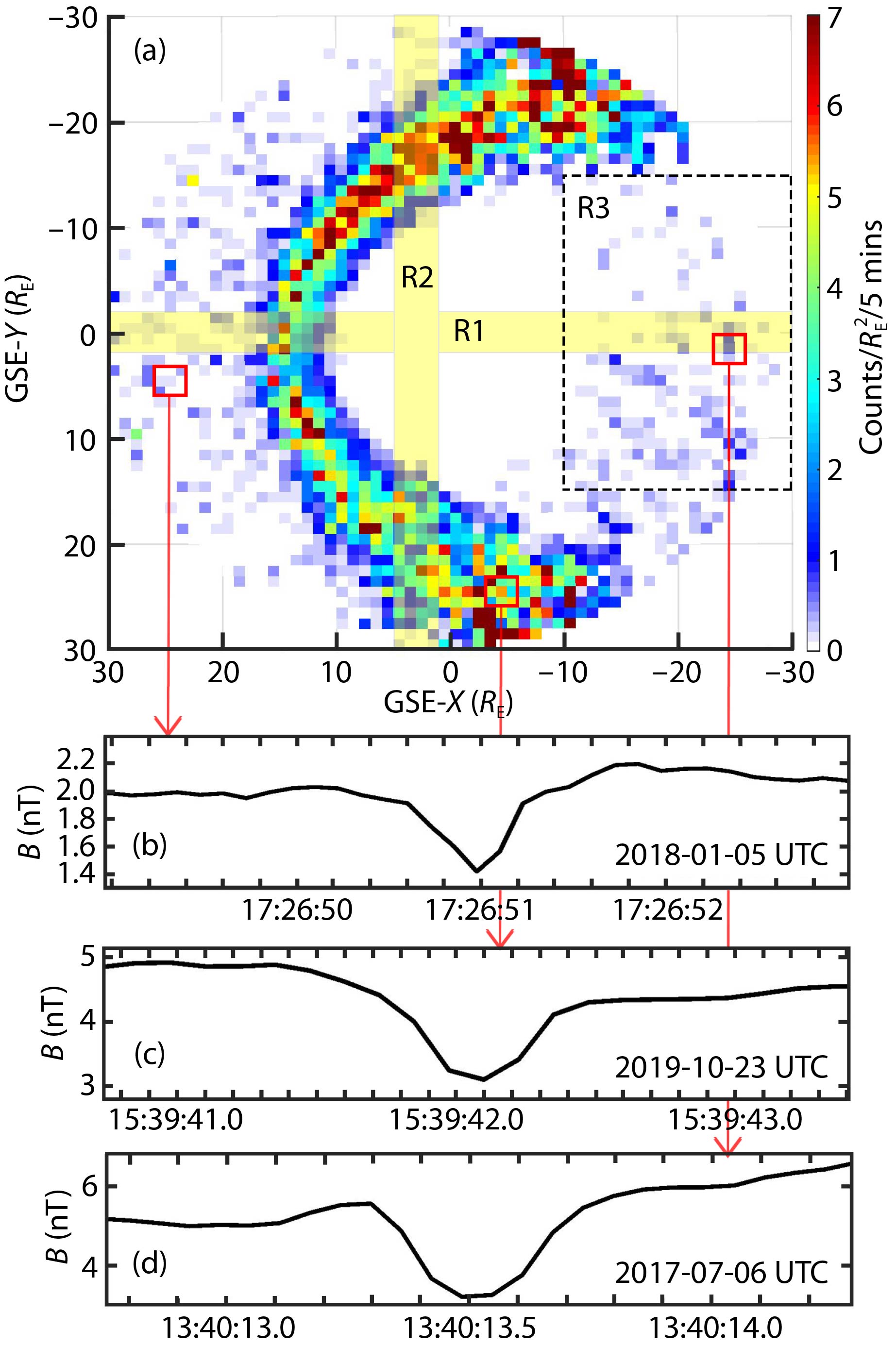
An automated routine is used to select KSMHs by computing the average and minimum magnetic field strengths, $|{B}_{\rm{ave}}|$ and $|{B}_{\rm{min}}|$, within a time window of 5 s. Events with $|{B}_{\rm{min}}| /|{B}_{\rm{ave}}| \le 0.75$, and $\omega \le 15^ \circ$ are retained, where $\omega$ is the angle between the magnetic field vector averaged over a 1 s interval before and after the structure. Similar event selection criteria can be found in Zhang TL et al. (2008), Yao et al. (2017), and Huang SY et al. (2019). Furthermore, additional conditions are attached to ensure that the selected events are more likely to be KSMHs: 1) a relatively stable environment ($|{B}_{\rm{ave}}| /{\sigma }_{B}\ge 2$ and ${\sigma }_{B}\le 5\, {\rm {nT}}$, where ${\sigma }_{B}$ is the magnetic field standard deviation over the time window), 2) a significant magnetic field depression ($\left|{B}_{\rm{ave}}\right|-|{B}_{\rm{min}}| \ge {\sigma }_{B}$), and 3) a minimum time duration of the structure ($\Delta t\ge 0.125\,{\rm s}$, e.g., the structure is identified by at least two data points, where $\Delta t$ is the structure duration when $B\le {B}_{\rm{ave}}-{\sigma }_{B}$). Approximately 0.2 million (192, 655) events were selected under these criteria over ~1672 days of available MMS data.

3.   Statistical Results and Analysis

3.1   Occurrence Rate

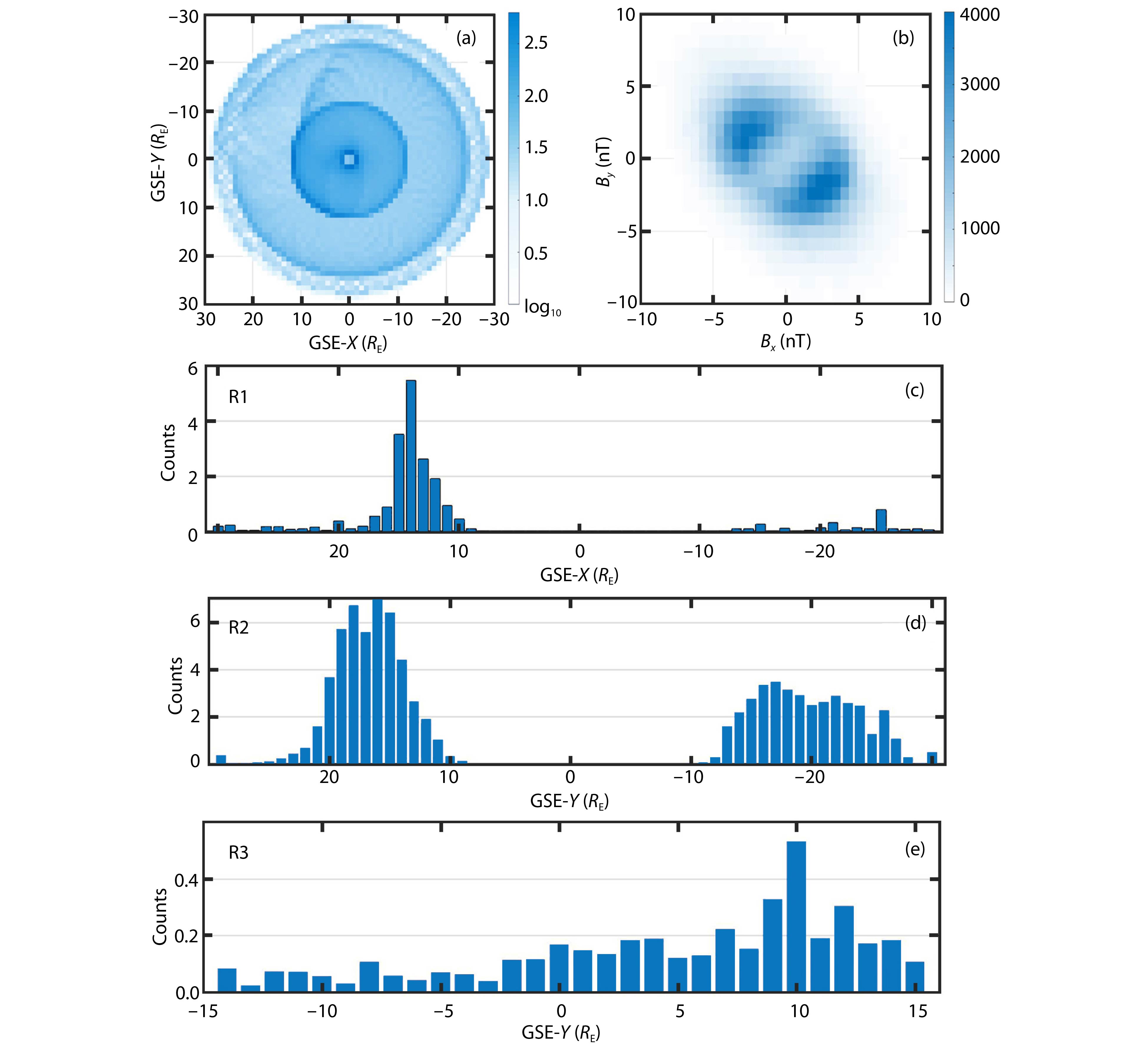
For the statistical study of the occurrence rate of KSMHs in terrestrial space, one important issue is to take into account the amount of time the satellites spend in a given region of space. That is, a high number of events in some regions may be caused by repeated satellite passes through this region; on the other hand, a low number may be due to the fact that the satellites seldom passed there. It is therefore necessary to calculate the number of events normalized by the “orbit density”, which has not been done in previous studies. The orbital density is defined as the number of times (counts) the satellite remained within an area of 1R_E² of a given location (X_GSE, Y_GSE) for a duration of 5 minutes (Figure 3a). Figure 2a shows the number of KSMHs normalized by orbit density. The color for each bin in Figure 2a is the quantity of KSMHs observed by MMS in 1R_E² per 5 mins (counts/R_E²/5 mins) in the GSE X-Y plane. Three areas can be clearly identified: the solar wind, magnetosheath, and magnetotail. Three examples observed in these areas are shown below (Figures 2b-d). We mark three regions in Figure 2a (R1, R2, and R3) for quantitative comparisons. Region 1 is used mainly to compare the occurrence rate of KSMHs in the solar wind, magnetosheath, and magnetotail. Regions 2 and 3 can be used to compare the occurrence rate between the dawn and dusk sides in the magnetosheath and magnetotail. The average values of each region are shown in Figures 3c-e. Significantly, we find that the number of KSMHs observed in the magnetosheath is greater than that in the solar wind and magnetotail (Figure 3c). This demonstrates that most of the KSMHs observed in the magnetosheath are generated locally, instead of propagating into the magnetosheath along with the solar wind. It is worth noting that the structure motion speed is also an important factor. In other words, the faster the structure moves, the more structures the satellite could observe. Thus, the occurrence rate in Figure 2a is further normalized by the background plasma flow velocity, where we assume that the structure is non-propagating in the plasma flow. The result is consistent with Figure 2a and shown in the Supplementary Materials (Figure S1).

Figure  3.  (a) The orbital density, defined as the counts of 5-minute intervals in which a satellite is located within a 1R_E² (X_GSE, Y_GSE) rectangular bin. (b) Statistical results for the IMF conditions during each event. (c–e) Details for the regions marked in Figures 2a.

DownLoad: Full-Size Img PowerPoint

Figure  2.  (a) The occurrence rate of KSMHs normalized by the orbit density (Figure 3a). The color indicates the number of observed KSMHs in 1R_E² per 5 mins (counts/R_E²/5 mins). Examples in the solar wind, magnetosheath flank, and magnetotail are shown in (b–d). Three regions (R1, R2, and R3) are marked for quantitative comparisons, and details can be found in Figures 3 (c–e).

DownLoad: Full-Size Img PowerPoint

From Figures 2a and 3d, one can find that there are more KSMHs in the dawnside magnetosheath than in the duskside. It is well known that the magnetosheath is more turbulent downstream of the quasi-parallel shock (Q_∥) than it is behind the spatially extended quasi-perpendicular shock (Q_⊥) (e.g., Lucek et al., 2005). We examined the interplanetary magnetic field (IMF) conditions for each event in our study. Most of the time, the IMF configuration can be considered to be that of a Parker spiral (Figure 3b). Thus, the dawnside magnetosheath can be roughly considered as downstream of Q_∥, and is more turbulent than the duskside magnetosheath. The statistical results in Figure 2a and Figure 3d are consistent with the argument that KSMHs tend to be generated in more turbulent environments. Furthermore, a significant dawn-dusk asymmetry (duskside dominating) is found in the magnetotail (Figure 3e). This feature has been discussed by Huang SY et al. (2019); however, since MMS burst mode data were used, few events were observed in their study. The statistical results normalized by the orbit density in our study are consistent with and confirm the findings of Huang SY et al. (2019).

Figure 4 displays the statistical results of 1-min-averaged environmental parameters (magnetic field and ion velocity) for the KSMH events in region R3. One can observe that the distributions of the magnetic field on the dawnside and duskside are similar; however, there are significant differences in event counts (Figure 4a-c). The X_GSE and Y_GSE components of the magnetic field (B_x, B_y) are distributed nearly symmetrically around zero (Figure 4a, b), and the Z_GSE component of the magnetic field (B_z) is mainly positive (Figure 4c, 89.5% on the dawnside and 83.3% on the duskside). Generally, B_z within the magnetotail plasma sheet is nearly northward because the magnetic fluxes emanate from the southern hemisphere and converge into the northern hemisphere. However, southward (negative) B_z can also be observed in the plasma sheet because of magnetic reconnection (e.g., Øieroset et al., 2001), flux ropes and plasmoids (e.g., Slavin et al., 1995), and magnetic disturbances caused by current disruption (e.g., Lui, 1996). Hence, to some extent, areas where magnetic activity occurs can be indicated by southward B_z. In our study, although the distributions of B_z on the dawnside and duskside are similar, there is still a significant difference in negative B_z between the duskside (16.7%) and dawnside (10.5%). This indicates that the dawn-dusk asymmetry of KSMHs in the magnetotail may be related to magnetic activity regions. Furthermore, the average value of positive B_z on the duskside (2.6 nT) is less than on the dawnside (3.6 nT), implying that the duskside magnetic field curvature radius is smaller and its current sheet is thinner (e.g., Büchner and Zelenyi, 1989; Rong ZJ et al., 2011). This thin current sheet is considered to be generated by a stronger Hall effect on the duskside (e.g., Lu S et al., 2019). Huang SY et al. (2019) also suggested that the dawn–dusk asymmetry of KSMHs in the magnetotail could be caused by the Hall effect. In Figure 4d, we find that the velocity in the X_GSE direction (V_x) is mainly Earthward (77.6% on the dawnside and 69.5% on the duskside). Furthermore, 36.1% and 22.8% of these events have velocities greater than 300 km/s and 400 km/s, respectively. This indicates that some events are related to magnetic reconnection, which is an important mechanism for producing high-speed flows in Earth’s magnetotail. In Figure 4f, the velocities of the Z_GSE component (V_z) are distributed similarly between dawnside and duskside. Significantly in Figure 4e, the velocities of the Y_GSE component (V_y) in dawnside are distributed symmetrically around V_y = 0, while a greater proportion (70.1%) of events on the duskside have V_y > 0. It can be seen that the velocity of events whose counts on the duskside exceed the dawnside are mainly distributed in V_y > 0 (89.2% of the total difference). This indicates a close relationship between the KSMHs and V_y. However, based on the current data, we cannot draw a conclusion with any certainty about the underlying physical processes involved. If the dawn-dusk asymmetry is caused by the near-Earth flow deflection, it indicates that a possible relationship with positive V_y, because the distribution of V_y for the dawnside events is basically symmetric. Another possible scenario is related to the location of magnetically active regions. For example, activities such as magnetic reconnection are more frequent on the duskside, and may generate more KSMH events that are observed in the deflection flows by spacecraft.

Figure  4.  Statistical results of 1-min-averaged environmental parameters (magnetic field and ion velocity) for the KSMH events in region R3.

DownLoad: Full-Size Img PowerPoint

3.2   Scales and Depths

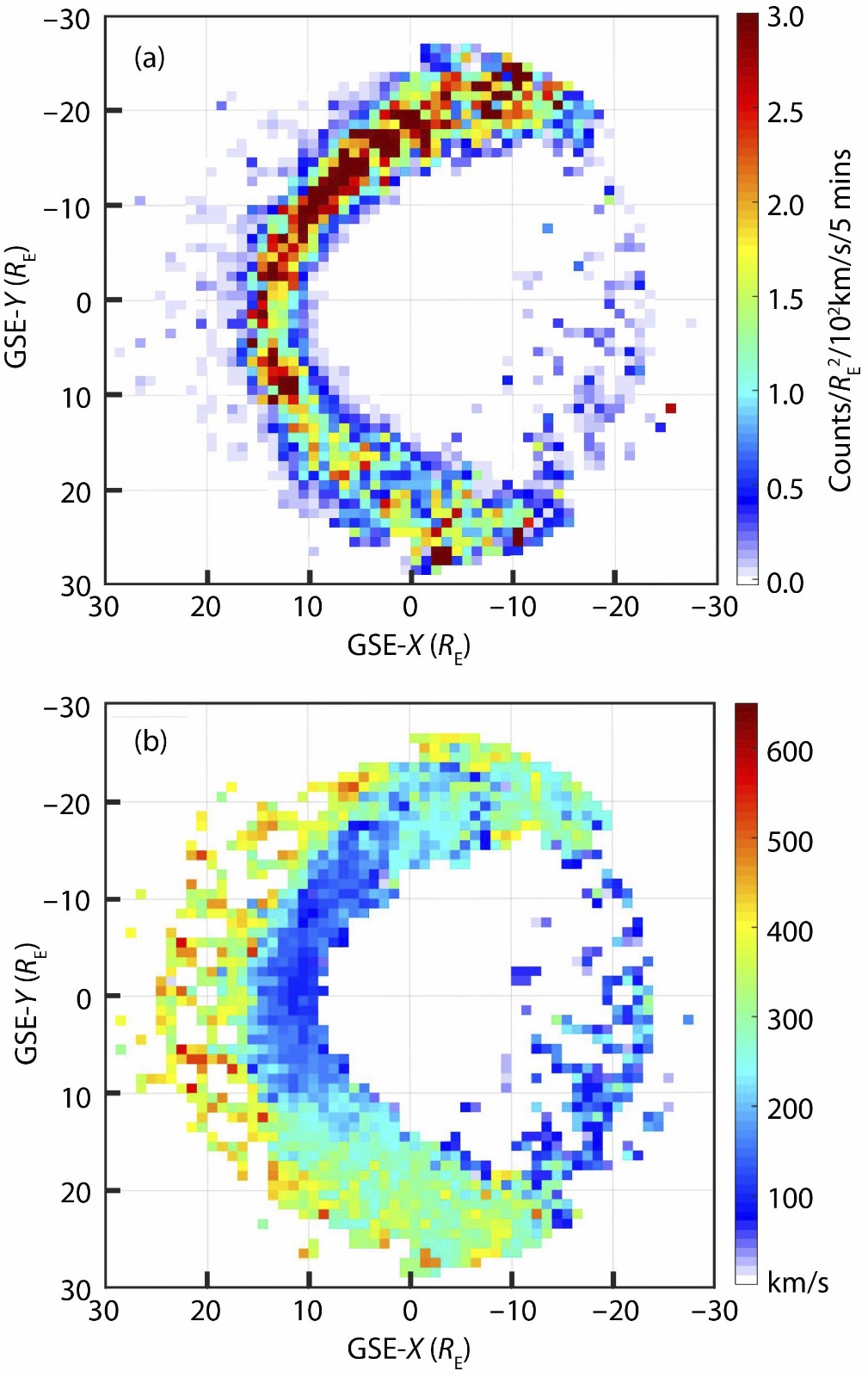
To uncover the characteristics of the magnetosheath KSMHs, the scales and depths of the structures are studied. Machine learning is used to identify the duration of MMS located in the dayside magnetosheath proper (Freund and Schapire, 1997). These magnetosheath time intervals are chosen to be longer than 5 mins in duration and limited to instances when MMS was in the dayside (Rx > 0). The KSMHs belonging to these periods are selected (in total 78, 503 events), and the average value of their scales and depths in each (X_GSE, Y_GSE) bin are shown in Figure 5a-c. The spatial-scale is calculated from ${V}_{\rm i} \cdot \Delta t/{\rho }_{\rm i}$, assuming the structure is propagating with the plasma flow, where V_i is the 1-min-averaged ion bulk velocity of MMS1, and Δt is the structure duration (temporal–scale). The ion gyroradius ρ_i is calculated from 1-minaveraged magnetic field and ion temperature measurement of MMS1. Figure 5a shows that the temporal–scales of the KSMHs increase when close to the magnetosphere, and Figure 5b shows that the spatial-scale near the subsolar magnetosheath is smaller than that in the flanks. Figure 5c plots the depth ($\left(1- \left|{B}_{\rm{min}}\right|/ \left|{B}_{\rm{ave}}\right|\right)\times 100{\%}$) of the KSMHs and suggests that the KSMHs become shallower from the bow shock to the magnetopause; however, this feature is not very obvious. The plasma beta and Mach number are also important parameters for the investigation of KSMHs. For example, if the bow shock is a Q_⊥ shock, the high beta and Mach number region is more turbulent in the magnetosheath. Here we show the statistical results of the beta and Mach number in Figure 5d and 5e. One can see that the distributions of beta and Mach number are basically symmetric in the magnetosheath, suggesting that they may not play a major role in the asymmetric KSMH occurrence rate between the regions downstream of Q_∥ and Q_⊥. The value of beta in the subsolar magnetosheath is greater than in the flanks, while the opposite is the case for the Mach number. This is basically as expected for the physical processes occurring in the magnetosheath. Considering the compression of magnetic fluxes in different regions, and that the scale is smaller when it is normalized by ρ_i in a high beta environment, we can infer that the KSMHs appear to be compressible. The available data, however, do not provide a definitive analysis. A more detailed analysis is necessary — for example, to calculate and normalize the distance from each event to the bow shock and magnetosphere, and to compare the position of each event with its characteristics. Another possibility that could be tried in the future would be to normalize the structure location using a 2-D model of solar wind plasma flow/convection around the magnetopause and carry out the analysis based on streamlines.

Figure  5.  The averaged parameters in each bin for the KSMHs in the magnetosheath proper.

DownLoad: Full-Size Img PowerPoint

Here we also need to discuss the uncertainty of the spatial and temporal scales. Since the temporal–scale ($\Delta t$) is the duration when the magnetic field strength is lower than $\left|{B}_{\rm{ave}}\right|$, the uncertainty of $\Delta t$ has been taken into account in the criterion that $\left|{B}_{\rm{ave}}\right|-|{B}_{\rm{min}}| \ge {\sigma }_{B}$, where ${\sigma }_{B}$ is used to represent the uncertainty of $\left|{B}_{\rm{ave}}\right|$. That is, the uncertainty of $\Delta t$ is the duration when the magnetic field strength is between $\left|{B}_{\rm{ave}}\right|-{\sigma }_{B}$ and $\left|{B}_{\rm{ave}}\right|+{\sigma }_{B}$. Because we need to make sure that the selected duration is indeed the duration of the structure, the lower limit of uncertainty is used in this study ($\left|{B}_{\rm{min}}\right|\le \left|{B}_{\rm{ave}}\right|-{\sigma }_{B}$). For the spatial-scale, the uncertainties come from the ion bulk velocity, magnetic field, and ion temperature. The standard deviations of these parameters over 1 min were calculated for the events, and used to calculate the uncertainty in spatial-scale. We find that 68.3%, 87.2%, and 93.4% of the events have uncertainties less than 10%, 20%, and 30%, respectively. This indicates that most of the spatial–scale uncertainties of the events are small and do not affect the results.

4.   Summary and Discussion

In this study, KSMHs detected by MMS in near-Earth space are statistically analyzed. The main results are:

(1) The occurrence rate of KSMHs in the magnetosheath is far above that in the solar wind.

(2) The occurrence rate of KSMHs downstream of Q_∥ is higher than that of Q_⊥.

(3) The occurrence rate of KSMHs in the magnetotail exhibits a dawn-dusk asymmetry (duskside dominating).

(4) The temporal-scales of KSMHs increase as their depth is decreased when close to the dayside magnetopause.

(5) The spatial-scales of KSMHs near the subsolar magnetosheath are smaller than in the flanks.

These results indicate that most of the KSMHs in the magnetosheath are generated locally, rather than advected with the solar wind. The asymmetry of the occurrence rate between the downstream of Q_∥ and Q_⊥ is important evidence that KSMHs tend to be generated in more turbulent environments.

Studying the evolution of KSMHs is challenging. Their small scale and short duration make it difficult to investigate their structure by satellite observations. For example, it is hard for two satellites to observe the same structure at different times unless they are extremely close together. For satellite constellations (with e.g., MMS and Cluster), it is therefore hard to know whether the observations given by different satellites indicate temporal evolution or spatial-variations of observed structures. To study this issue, one possible way is to investigate statistically their structure properties and their relation to the background plasma environment. From Figure 5b one can observe that the structures’ spatial–scale at the flanks are larger than when they are observed near the subsolar magnetosheath. It is known that the pressure near the subsolar magnetosheath is higher than in the flanks. A possible scenario is that the structure's spatial–scale is affected by its local pressure environment. A previous study has found that pressure variation can significantly affect how structures contract or expand, affecting their scale (Yao ST et al., 2020a), which supports the above deduction. That said, the spatial-scales near the magnetopause downstream of Q_⊥ are larger than in other positions — an observation that is worthy of further study.

Supplementary Materials

  S1.  (a) The occurrence rate of KSMHs normalized by the orbit density and structure velocity. Here we assume that the KSMHs are non-propagating in the plasma flow, and use 1-min-averaged background plasma flow velocity (b) instead of structure velocity. The color indicates the number of observed KSMHs in 1R_E² per 10² km/s per 5 mins (counts/ R_E²/10² km/s/5 mins).

DownLoad: Full-Size Img PowerPoint

Acknowledgments

We thank the MMS and AMDA teams for providing science data (https://lasp.colorado.edu/mms/sdc/public/; http://amda.cdpp.eu/). This work was supported by the National Natural Science Foundation of China (grants 41731068, 41774153, 41941001, 41961130382, 41431072, and 41704169) and Royal Society NAF\R1\191047. We thank the International Space Science Institute (ISSI) for their support. Z. H. Y. is supported by the PRODEX program managed by ESA in collaboration with the Belgian Federal Science Policy Office.